Volume of a Spherical Cap Calculator
Spherical Cap Shape
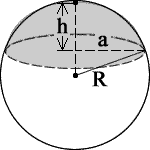
R = sphere radius
a = cap base radius
h = height of cap
V = volume
S = surface area
π = pi = 3.14159
√ = square root
Calculator Use
Online calculator to calculate the volume of geometric solids including a capsule, cone, frustum, cube, cylinder, hemisphere, pyramid, rectangular prism, sphere and spherical cap.
Units: Note that units are shown for convenience but do not affect the calculations. The units are in place to give an indication of the order of the results such as ft, ft2 or ft3. For example, if you are starting with mm and you know a and h in mm, your calculations will result with V in mm3.
Below are the standard formulas for volume.
Volume Formulas:
Capsule Volume
- Volume = πr2((4/3)r + a)
- Surface Area = 2πr(2r + a)
Circular Cone Volume & Surface Area
- Volume = (1/3)πr2h
- Lateral Surface Area = πrs = πr√(r2 + h2)
- Base Surface Area = πr2
- Total Surface Area
= L + B = πrs + πr2 = πr(s + r) = πr(r + √(r2 + h2))Circular Cylinder Volume
- Volume = πr2h
- Top Surface Area = πr2
- Bottom Surface Area = πr2
- Total Surface Area
= L + T + B = 2πrh + 2(πr2) = 2πr(h+r)Conical Frustum Volume
- Volume = (1/3)πh (r12 + r22 + (r1 * r2))
- Lateral Surface Area
= π(r1 + r2)s = π(r1 + r2)√((r1 - r2)2 + h2)- Top Surface Area = πr12
- Base Surface Area = πr22
- Total Surface Area
= π(r12 + r22 + (r1 * r2) * s)
= π[ r12 + r22 + (r1 * r2) * √((r1 - r2)2 + h2) ]Cube Volume
- Volume = a3
- Surface Area = 6a2
Hemisphere Volume
- Volume = (2/3)πr3
- Curved Surface Area = 2πr2
- Base Surface Area = πr2
- Total Surface Area= (2πr2) + (πr2) = 3πr2
Pyramid Volume
- Volume = (1/3)a2h
- Lateral Surface Area = a√(a2 + 4h2)
- Base Surface Area = a2
- Total Surface Area
= L + B = a2 + a√(a2 + 4h2))
= a(a + √(a2 + 4h2))Rectangular Prism Volume
- Volume = lwh
- Surface Area = 2(lw + lh + wh)
Sphere Volume
- Volume = (4/3)πr3
- Surface Area = 4πr2
Spherical Cap Volume
- Volume = (1/3)πh2(3R - h)
- Surface Area = 2πRh
Triangular Prism Volume
\[ V = \dfrac{1}{4}h \sqrt{(a+b+c)(b+c-a)(c+a-b)(a+b-c)} \]